Answers:
1 (a)

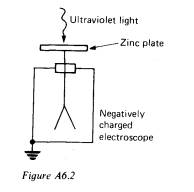
You might describe an experiment in which a clean negatively charged zinc
plate is illuminated with ultraviolet light and the charge loss due to the
emission of electrons is detected by means of an electroscope connected to the
zinc plate. This is shown in Figure A6.2.
(b) (i) The term photon is used to describe the
quantum of energy, the packet of energy, of electromagnetic radiation.
(ii) The work function W is the energy needed to remove, an electron from
the metal concerned.
(iii) The number of photons incident on the metal surface per second
is directly proportional to the intensity of the radiation. Each photon may
eject an electron and so the number of electrons emitted is directly
proportional to the intensity.
(iv) The
energy E carried by a photon is directly
proportional to its frequency f and is not dependent on the number of photons
incident on the surface per second:
E=hf
where h is the Planck constant. A photon collides with an electron
within the metal and transfers energy to it. Those electrons at the surface are
emitted with the energy given to them by the photons minus just the work
function W; they thus have maximum velocity. Electrons below the surface of the
metal 'use' more energy than W in escaping from the metal and so emerge with a
lower velocity. There is thus a range of velocities from zero up to a maximum.
The maximum velocity v. is given by
E= hf = ½
mv2max + W
Increasing the
frequency of the radiation increases the energy of the photons and so increases
the velocities of the electrons.
(v) No emission can occur if the energy of a photon is less than the
work function. Thus as the energy of a photon is hf there is a minimum frequency below which
emission cannot occur. This minimum is given by
hf
= W
(e) Photoelectric cells are used in a variety of applications where
objects have to be counted automatically. Objects can be moving along a conveyor
belt with a beam of light passing at right angles to the conveyor belt to a
photocell. When an object moves along the belt it interrupts the light beam and
so gives rise to a change in the photocell current. This change in current can
be used to activate a counter.
There are
many other applications you might prefer to describe, e.g. the reproduction of
sound from the sound-track on a cine film, a burglar alarm where a light
(infrared) beam is interrupted, a light meter in photography.
(b) (i) The photoelectric effect and Einstein's interpretation of it in terms of the quantization of electromagnetic radiation is an obvious piece of experimental evidence. The main points that would need to emerge in a discussion of it is that:
1. No electrons are emitted from a metal when the radiation has a frequency lower than a certain threshold value, this value depending on the metal concerned.
2. The kinetic energy of the emitted electrons varies from zero up to a maximum value, this maximum depending only on the frequency of the radiation and being independent of the intensity of the radiation.
3. The number of electrons emitted per second, with monochromatic light, is proportional to the intensity of the radiation.
4. Emission of electrons occurs effectively instantaneously when the metal is illuminated; no time lag occurs.
The quantization of the electromagnetic radiation such that the energy of a quantum is equal to E=hf
where f is the frequency and h the Planck constant, enables the above experimental results to be explained.
If the energy of the incident quantum, the photon, is not high enough, i.e. if it does not have a high enough frequency, then insufficient energy is given to an electron in the metal to enable it to escape.
energy of photon = energy W needed to remove electron + kinetic energy given to electron
The energy of the photon has to be greater than W for emission to occur. The energy needed to remove an electron from the metal is the smallest for electrons at the metal surface and greater for electrons further below the surface. Thus the kinetic energy of the emitted electrons varies from zero to a maximum, this occurring for the electrons at the metal surface. Increasing the intensity of the radiation does not change the energy of a photon, and hence has no effect on the energy of the emitted electron. It does, however, change the number of photons hitting the surface per second and so does change the number of electrons emitted per second. The effectively instantaneous emission of the electrons can be explained only if the energy of the radiation is quantized. A spread of the energy over a wavefront would mean that a significant time would have to elapse before sufficient energy arrived for emission to occur.
The distribution of thermal radiation with wavelength from a black body presents a problem for an explanation based on electromagnetic wave theory but is capable of explanation in terms of the quantization of electromagnetic radiation. It was this problem which can be considered to be the start of quantum theory.
(ii) For the electrons,
p = mv
= 9.0 X 10- 31 x 5 x 106 = 4.5 x 10-24 kg m s-1
Hence they will have a wavelength l of
6.6 x 10-34 / (4.5 x 10 – 24) = 5 x 10-10 m
This is a wavelength of the same order as the spacing between planes in a crystal. Thus diffraction of a beam of electrons by a crystal could lead to a demonstration of the wave properties of electrons. In 1927 C. Davisson and L. H. Germer found that when a beam of electrons was directed at a crystal the results showed intensity variations just like those obtained for X-rays reflected from crystals.